Speaker
Description
AMR magnetometers are used in many applications where low power consumption is desired, particularly in space applications [1] where it is one of crucial parameters. Although fluxgate/optically pumped magnetometers dominate space industry due to their ultralow-noise, AMRs are increasingly used if the roughly 100-fold increase of noise is not an issue, as they are miniature, lightweight, solid state and readily available (off-the-shelf). [2] In this work, we propose a novel method for reducing power consumption while maintaining good precision/low noise of the instrument. For the best precision and stability, AMR magnetometer almost always utilizes feedback compensation and so-called flipping (set/reset) technique. Both greatly increase power consumption. Flipping power consumption can be reduced by utilizing ultrashort pulses [2] generated by MOSFET H-bridge instead of traditional capacitor coupled half bridge. Closed loop magnetic field compensation leads to great improvement in linearity of magnetometer and its temperature stability of sensitivity. However, in many applications, measured magnetic field is steady and its variation is slow or with only occasional fast perturbations. The same applies also for temperature of magnetometer itself. In our contribution, we propose a method where we switch from open-loop operation into feedback (closed-loop) only when necessary. With a fast feedback-loop settling, power consumption can be greatly reduced: on tested magnetometer with HMC1021, compensation in closed loop requires approx. 100 mW for steadily compensating 50 $\mu$T field, but only 10 mJ per correction measurement. Proposed algorithm uses three input variables to decide if “calibration” measurement with closed loop is necessary - namely: change in temperature, change in magnetic field and time elapsed from last calibration. After closed-loop “calibration” is performed, open-loop measurement is used as an additional ΔB to the last closed loop absolute measurement. In Fig 1., a slowly rising magnetic field was applied, algorithm action can be seen as a correction (when $\Delta B$ > 1 $\mu$T). Impact on linearity of magnetometer using proposed algorithm can be seen in Fig 2. (where criterium for calibration is set to $\Delta B$ > 5 $\mu$T).
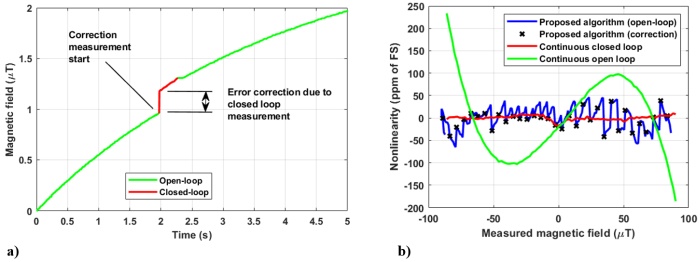
Fig. 1 a) Response of algorithm to rising magnetic field. b) Linearity comparison (algorithm, OL, CL).
Acknowledgements
This work was supported by the Grant Agency of the Czech Technical University in Prague, grant No. SGS22/170/OHK3/3T/13.
References
[1] M. Díaz-Michelena, “Small Magnetic Sensors for Space Applications,” Sensors, vol. 9, no. 4. MDPI AG, pp. 2271–2288, Mar. 30, 2009. doi: 10.3390/s90402271.
[2] Leitner et al., “Design of the Magnetoresistive Magnetometer for ESA’s SOSMAG Project,” IEEE Transactions on Magnetics, vol. 51, no. 1. Institute of Electrical and Electronics Engineers (IEEE), pp. 1–4, Jan. 2015. doi: 10.1109/tmag.2014.2358270.

